Copyright ©2009 by Paul Niquette. All rights reserved. |
|
|
|
|
 light is
distinguished from other modes of transportation in
several important -- well, ways.
Railroads are confined to trackways, buses roadways,
and ships seaways (all right, sea lanes).
Whereas aviation has long been confined to airways,
with the advent of GPS
technology, flights are increasingly being allowed to
fly direct routings by air traffic control (ATC).
In and of itself, that will become a boon to
conservation in the sky (for small values of
"boon"). Altitudes, the third dimension in airways,
must still be assigned to each flight in proximity to
others. Same for airspeed along common pathways.
Finally, in varying ways, even the steepness of
ascent and descent must be prescribed by ATC. All
for safety.
Setting aside these constraints for now, let us address the question in the abstract, using the three fundamental phases of flight as outlined in the puzzle: [Phase 1] ascend to cruise altitude, [Phase 2] cruise in level flight, and [Phase 3] descend for the approach to landing. Taking the easy one first... [Phase 2] Cruising in Level Flight All public transportation vehicles that engage in motion through air -- buses, planes, trains -- must overcome a force called 'drag', which tends to retard motion. Airplanes are distinguished in that for them, there are two kinds of drag. Common old ordinary wind resistance is called 'parasite' drag, which becomes greater according as the square of airspeed. Airplanes also experience a curious form of drag, which paradoxically increases at low airspeeds. It is called 'induced' drag -- induced by the act of flying (see Wages of Flight). Here is a schematic diagram of drag...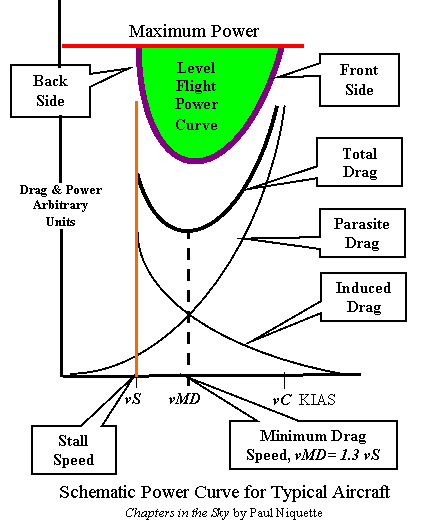 
A fully loaded airliner with flaps up and landing gear retracted will stall at vS = 175 knots (201 MPH). Thus, for Green Flight, the airliner would cruise at vMD = 228 knots (262 MPH). Nota bene: That is still faster than the fastest bullet trains in service.For fastest flight, a typical jet airliner would have a cruise speed vC of, say, 500 knots (575 MPH), which is 120% over its minimum drag speed vMD... vC = 2.2 vMDStarting at v = 0, parasite drag dP increases according as the square of speed... dP@v = K v2At vC, parasite drag dP@vC dominates the resistance to motion, allowing us to estimate that... dP@vC = K (vC)2Flying at vMD, parasite drag dP@vMD is about equal to induced drag dI@vMD so the total drag at vMD will be given by... dTotal@vMD = 2 dP@vMD = 2 K (vMD)2...allowing us to calculate the ratio... dP@vC / dTotal@vMD = K (vC)2 / 2 K (vMD)2 = (vC)2 / 2 (vMD)2  orsepower, of course, is what keeps the
plane aloft in level flight, by producing thrust,
which overcomes drag. Horsepower H varies
with the product of drag and speed, in other words, H
increases with the cube of speed (see the
green area in the figure above). The ratio of
horsepower required at vC over that at
vMD will be given by...
H@vC/ H@vMD= (vC)3 / 2 (vMD)3 = (2.2 vMD)3 / 2 (vMD)3 = 5.3Thus our typical airliner takes 5.3 times as much horsepower to fly only 2.2 times faster (500 knots vs 228 knots). Putting it the other way 'round, the effective "mileage" for Green Flight at vMD is 2.4 times that at vC, which is an immense savings in both energy and carbon footprint. An exclamation point may be warranted for the previous sentence. [Phase 3] Descent for Approach to Landing. Horsepower is not needed. With engines idling, an aircraft will glide at an angle of descent determined by the aerodynamic lift-to-drag ratio. At minimum drag speed vMD the greatest distance can be covered while descending from cruise altitude. At zero power, accordingly, our Green Flight enjoys an infinite mileage. Until it runs out of altitude AGL, of course. [Phase 1] Ascent to Cruise Altitude. Here again, flying at minimum drag speed vMD means the greatest distance can be covered using the least amount of fuel. That leaves any extra horsepower available for the climb (see the green area in the sketch above). Greater the extra horsepower the faster the climb -- vertically -- and the sooner the flight reaches cruise altitude. At any given indicated airspeed, by the way, the higher the altitude, the faster the true airspeed. Getting to cruise altitude quickly improves the performance of the Green Flight. The faster the rate of climb the more horsepower will be demanded. Following whatever rate of climb, though, the aircraft's cruise altitude constitutes potential energy. In [Phase 3] we observed that during descent, potential energy repays much of the energy expended for the climb. Nota bene: "Power" and "energy" are different things (see Erg and Ugh).Accordingly, the maximum amount of horsepower available for climbing at vMD might be estimated from the expression developed from [Phase 3] as about 80%. H@vC / H@vMD = 5.3  One might suppose that a typical
airliner in the future will be fitted with
considerably smaller engines for Green Flight than for fast flight. However,
quick climbing to cruise altitude demands peak
horsepower. So too does acceleration on the
runway for take-off and deceleration during the
landing roll. Engines won't get smaller, but
maybe fuel tanks will. |
|
NOTE: Taking into account winds aloft -- particularly headwinds -- does change the calculation. This challenge is addressed in a puzzle entitled Wind Circle. |
orresponding to the bottom of the curve
one finds the "minimum drag speed" vMD,
which is usually about 30% faster than the
airplane's stall speed vS. In
still-air, vMD is the most efficient
airspeed, giving the greatest distance for the least
fuel consumed. Think MPG. We now answer
the question in the 