|
Orbital Deflection |
|||
|
Research
programs throughout the worldwide community of
nations have resulted in various proposals for
avoiding impacts by Near Earth Objects
(NEOs). For success, they must addressing the overall
requirements for Detection, Prediction, and
Deflection.
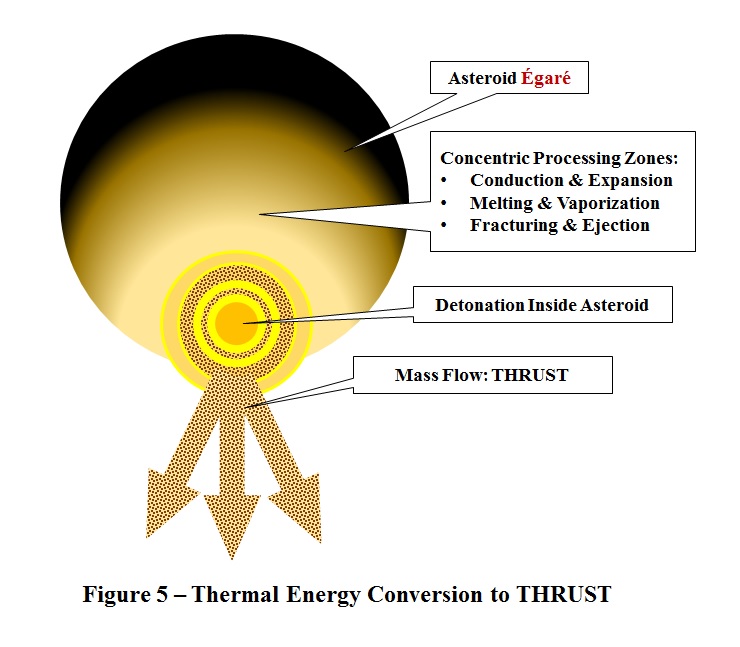
Solvers saw in Figure 4 that simply setting
off a thermonuclear blast on the surface of Égaré
throws away most of its energy, and what's left
irradiates the asteroid but does not show a whole
lot of competence in the vectored-thrust
department. Thus, we have concluded that our
objective comes down to using part of the asteroid's
own mass to produce the thrust. Placement of Energy Source Obviously,
placing the thermonuclear explosion inside
asteroid Égaré would
be far more effective in discharging mass into
space thereby generating thrust.
Just imagine...
Of
course, the expression 'effective yield' pertains
particularly to the Nuclear
Bunker Buster, which is designed to deliver
a nuclear warhead underground to destroy military
targets that have been buried in -- well,
'bunkers' -- and hardened by reinforced concrete
structures.
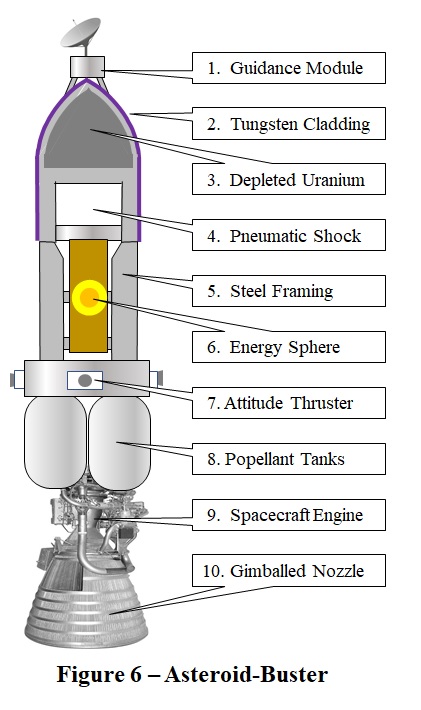
Solvers will find several references
with proposed features for a hardened penetrator,
from which we have made a few design choices for
the Asteroid-Buster, as
a theoretical spacecraft... 3. Depleted Uranium (D-38)
for inertial ballast inside the penetrator. 8. Propellant Tanks must be sized
for sufficient cryogenic
supplies to complete post-boost orbiting maneuvers
plus rendezvous, station-keeping and final
asteroid Égarpenetration
burn.
The
length of section 4 Pneumatic Shock may actually be quite
significant in determining the ultimate depth of
the thermonuclear blast.
Final Spacecraft
Maneuvers 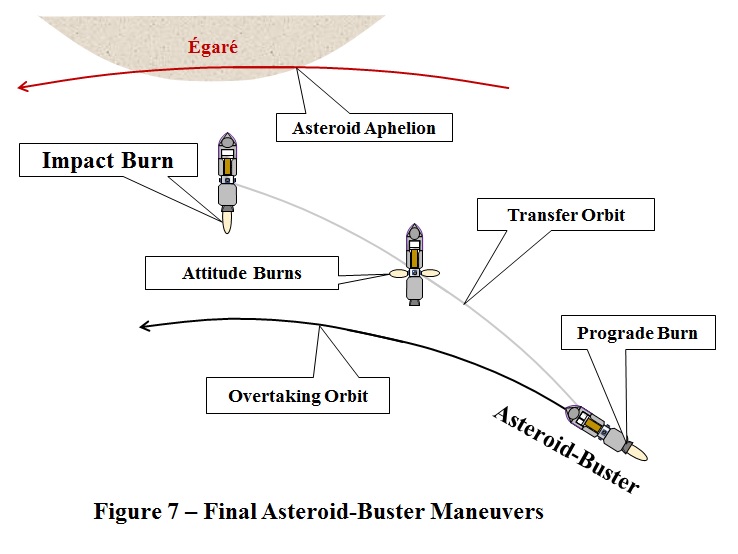
That will necessitate delivering the
spacecraft from Earth to an orbit inside
that of Égaré
with the same eccentricity -- but slightly behind
Égaré
in phase. With its shorter orbital period,
the satellite will gradually catch up to the
asteroid. At precisely the right moment
a ∆V Tangential
burn must be autonomically administered
to put the spacecraft into a Transfer Orbit.
Finally, the spacecraft must be repositioned by
Attitude Burns below the orbital plane, then
turned toward the asteroid for the ∆V Normal Impact Burn. Model for Proposed
Solution To
the question posed by the Orbital Deflection puzzle poses this
question...
...to which we have provided one answer in narrative
form.
Analyses with numerical specificity for the
orbital mechanics and the detailed design of Asteroid
Buster are
beyond the scope of this entry in Puzzles with a Purpose.
Nevertheless, in Figure 8 are a few elementary
tools that some solvers may find useful for
exploring these subjects further. 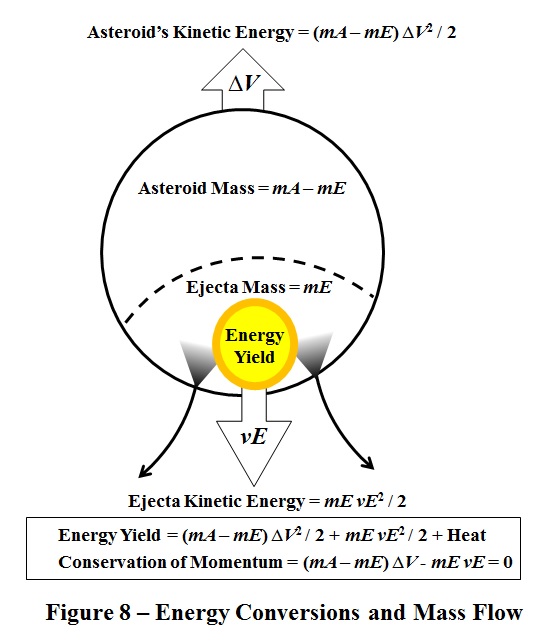
Let us
assume that the asteroid has uniform
density. Whatever the shape of the ablation
boundary inside the asteroid, proportionality
applies: mE
/ mA
= (dP
/ dA)3,
where… dA =
diameter of the asteroid; for Égaré, dA =
100 m. dP =
depth of the placement of
the thermonuclear device
∆V =
intentional change in asteroid’s velocity normal
to its orbital plane
hY = heat
energy yield
from the thermonuclear explosion, hL = heat
energy loss
eventually to space
...which is an estimate of net energy required for ∆V Normal and ∆i = 1/10
degree.
Base
Case and Perturbations
The calculations in the model formulated above can be regarded as a Base Case. Our estimate for the required thermonuclear energy (181 KtnTNT) needs to be tested for its sensitivity to the assumptions in the model. Thus we will 'perturb' three key parameters ↕10% and ascertain the consequences by re-running the model. Here is what is called a 'star diagram' for the results... 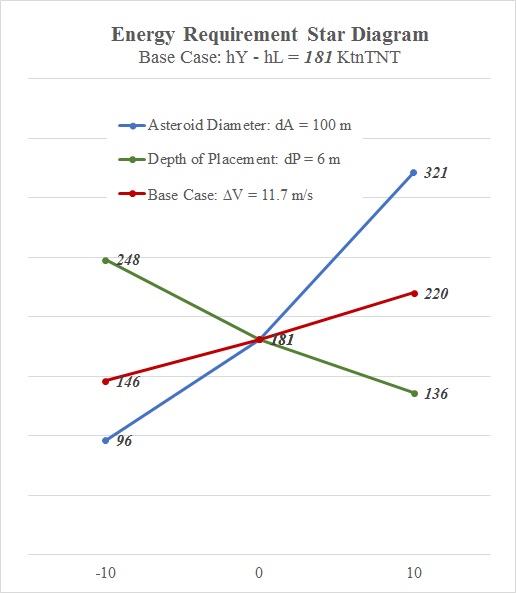
|