 o
calculate the exact value of the Golden Section
one needs to expressing the proportionalities in the
puzzle as ratios
which transforms into a simple quadratic equation...
...the solution to which solves the puzzle.
 =
|
For a line of, say, 100 centimeters, --
the Golden Section -- would measure
a little more than 61.8 centimeters, about 62% of the length of your line.
hat
number looks mighty familiar. Sure enough, from
a divided line, we have rediscovered  ,
the value towards which the ratio of two successive Fibonacci numbers tend,
as discovered two centuries ago by Robert Simson.
Here ancient number theory and aesthetics strangely join.
If the 100 centimeters mentioned above represents the vertical dimension
of a piece of fine art, then the artist might well have been advised to
position the focus of interest about 62 centimeters from the bottom. The
same theory influences the location side-to-side of artistic emphasis.
The viewer's eye will find that location most pleasing, according to adherents
of -losophy
(groan).
Thus do still-life artists place their baskets of fruit or
flowers at the Golden Section. Hah,
that desert scene: Did you happen to notice where the artist put the saguaro!
Look at the pictures around your room. Observe how often this 'rule' has
held.
The horizon-line in a seascape, for example. You should
expect to find it neither at the bottom of the canvas nor at the top --
not at the center, either. Coerced by the Golden
Section, the artist divides the canvas with sky-above-ocean
62% of the distance from the bottom, and breakers in the foreground thrust
upward an emphatic explosion of foam intersecting the horizon 62% of the
way from left or right.
The frame-maker, too, appears to answer the call of ,
imposing the 62% ratio, horizontal to vertical or vice versa. Ancient Greek
and Roman architects often designed their structures with the Golden
Section firmly in mind.
See for yourself: Take calipers to the Parthenon.
Curiously, all the artists known to the author retch at numerical
deliberations such as those set forth above. Nevertheless, they paint and
carve 62% proportions upon canvas and clay, responding presumably to private
mental maunderings not mathematical calculations.
 n
the dimensions of human face and figure, Golden
Sections preponderate. Check with that other Leonardo (da Vinci
-- not Leonardo da Pisa) on that. Some might attribute
such ratioing to Providential computations, others to Nature's aesthetic
intuition.
Such a relentless 'emergent property' must have prevailed
in many realms indeed. People think they observe 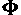in
relative sizes of successive chambers in the nautilus -- a seemingly natural
obedience to the ratio .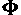
That may be going too far. Why should nature prefer 62%
as the annual growth factor for mollusks rather than some other? Sophisticated
persons are skeptical of claims
that are not based on closely reasoned arguments from first principles.
It is popular to see Fibonacci sequences and therefore
Golden
Sections...
-
in the spirals of sunflower heads,
-
in pine cones,
-
in leaf buds, and
-
in animal horns.
Oh yes, and don't forget, the elevation of the nominally
normal navel in proportion to the height of healthy humans (the belly button
of a 6-footer should be located almost exactly 44.5 inches above the floor).
Thus, a mystic with disproportionately long legs must be said to contemplate
a high-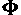 navel. Said
by whom? |
