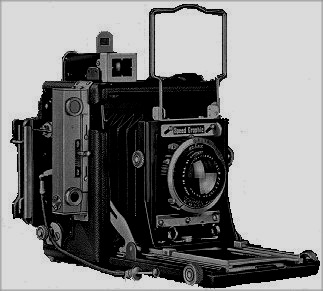
  Featured in the Historic Car Crash puzzle is a
photograph taken by a Speed
Graphic camera like this one,
which dates back to the 1930s. Look closely and
you will see a solenoid
mounted horizontally below the lens. It was
designed to be activated by a speedgun
unit for synchronizing the shutter with a flash
attachment (not shown in the photograph). Featured in the Historic Car Crash puzzle is a
photograph taken by a Speed
Graphic camera like this one,
which dates back to the 1930s. Look closely and
you will see a solenoid
mounted horizontally below the lens. It was
designed to be activated by a speedgun
unit for synchronizing the shutter with a flash
attachment (not shown in the photograph).
For the car-crash photograph, the
solenoid was activated by an electronic timer, which
was built in the laboratory of ITTE.
The
design of that timing device is quite a story in
itself, inasmuch as transistors,
let alone microprocessors,
were not commercially available in 1954.
An interested solver might like to try
his or her hand in the design of a monostable,
one-shot
multivibrator using a pair of vacuum tube triodes.
 A little
parsing music, please: Notice that the Historic Car Crash puzzle
does not seek a prediction of collision forces and
energy dissipation. ITTE's research, of course,
would give paramount importance to those topics, but
the puzzle merely calls for a time interval.
According to Engineered
Automobile Crashes a timer tripped the shutter
at a precise interval after the car contacted the
barrier in order to capture the instant of "maximum
deceleration and crush-in volume." That
phraseology, by the way, was specified by Derwyn Severy,
the project engineer. Both of those measurements
might pertain to the car itself. But what about
the second
impact and the collision forces that cause
injuries to people? A little
parsing music, please: Notice that the Historic Car Crash puzzle
does not seek a prediction of collision forces and
energy dissipation. ITTE's research, of course,
would give paramount importance to those topics, but
the puzzle merely calls for a time interval.
According to Engineered
Automobile Crashes a timer tripped the shutter
at a precise interval after the car contacted the
barrier in order to capture the instant of "maximum
deceleration and crush-in volume." That
phraseology, by the way, was specified by Derwyn Severy,
the project engineer. Both of those measurements
might pertain to the car itself. But what about
the second
impact and the collision forces that cause
injuries to people?
For the Historic Car Crash puzzle, let us reprise the 1954
analysis by the research assistant...
| He began
by noting that the time of maximum
deceleration Tmax-decel
experienced by the anthropometric dummies
would necessarily take place after the
time of maximum crush-in volume Tmax-crush for
the vehicle, thus Tmax-decel
> Tmax-crush. |
| Conservation of the vehicle's
kinetic energy seemed like a macrocosmic way
to estimating Tmax-crush, but it led into a blind
alley. With the weight of the vehicle W
and speed V both known, the kinetic
energy at impact was given by (1/2)(W/g)
V2. That energy would have to be
dissipated as heat and sound in the crunching
of the vehicle frame by an unknown force Fmax-crush
multiplied by Xmax-crush (see Observation #1) -- plus the
lifting of the vehicle's center of mass by
some Ymax-crush (see Observation #2) along with
whatever energy gets absorbed in the barrier
and the planet earth. All those
variables, even if perfectly known, were
useless in estimating an amount of time --
Tmax-crush. |
| A microcosmic analysis began
with a conventional equation that relates
stopping distance and stopping time:
Tmax-crush = 2 Xmax-crush / V. With V =
28 mph = 41 fps and Xmax-crush =
2.5 ft, the result was Tmax-crush =
0.061 seconds = 61 milliseconds. A
cursory review of the film from the first
crash test, even with frames separated by 25
ms, indicated that this time was too
short. No wonder. The calculation
made a hidden assumption:
constant deceleration. |
| A more realistic model for the
crash applied an elastic assumption
for the vehicle frame ("elastic" up to a point
-- the yield
point of the materials in the
structure). A metal, in particular
steel, acts pretty much like a spring,
resisting stress
in proportion to strain
until reaching its elastic limit. Stress
or force (f) during a collision
starts out at zero and increases with crush-in
displacement (x) linearly (f
= k x) until
reaching some maximum, such that f
= Fmax-crush and x = Xmax-crush,
after which f drops to zero and
x stays equal to Xmax-crush. |
| The deceleration of the
vehicle is given by d2x/dt2 = - (k / m)
x (Newton's
Second
Law), where m represents
the mass of the vehicle (m = W
/ g) and the minus sign indicates that the
collision force resists the inertia of the
vehicle, which will hardly surprise
anybody. |
Sophisticated solvers will recognize
the equation d2x/dt2
= - (k / m) x as the most
familiar homogeneous
second-order
linear, constant coefficient ordinary differential
equation, which is best known for its power to
describe simple harmonic motion (animation).
 The research
assistant's mathematical model, in effect, had a 1937
Plymouth 'vibrating' linearly back and forth in
obedience to x = Xmax-crush cos[(k
/ m)1/2 t], such that the period
is given by the following expression: 2 The research
assistant's mathematical model, in effect, had a 1937
Plymouth 'vibrating' linearly back and forth in
obedience to x = Xmax-crush cos[(k
/ m)1/2 t], such that the period
is given by the following expression: 2 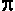 / ( k / m)1/2.
Well, of course, the vibration ceased after only
one-quarter of its period, with x = Xmax-crush, as the
car's structure stopped its "harmonic motion."
Accordingly, Tmax-crush = ( / ( k / m)1/2.
Well, of course, the vibration ceased after only
one-quarter of its period, with x = Xmax-crush, as the
car's structure stopped its "harmonic motion."
Accordingly, Tmax-crush = (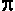 / 2) (m
/ k)1/2. / 2) (m
/ k)1/2.
Here may be a surprise for some people:
Tmax-crush is independent of Xmax-crush and
therefore independent of the speed of the vehicle V!
The exclamation point would have been an undeserved
indulgence at this point in the analysis, inasmuch as
the research assistant did not yet have an estimate
for Tmax-crush. Whatever that turns out to be,
though, it will be almost constant for a range of
crashing speeds and vehicle damage characterized by Xmax-crush.
Back in 1954...
| Finding the value of m
/ k required three more
assumptions...
[1] That the values of both m
and k are constant over the
range 0 < x < Xmax-crush.
[2] That 100% of the kinetic
energy of the vehicle is absorbed by the
vehicle.
[3] That an average Fmax-crush)
/ 2 prevails throughout the range 0 < x
< Xmax-crush.
|
As noted above the vehicle's
kinetic energy is given by (1/2)(W/g) V2.
Based on the assumptions, k = (W/g)
(V/ Xmax-crush)2 and Tmax-crush = (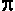 / 2) (Xmax-crush /
V). Accordingly, for Xmax-crush =
2.5 ft and V = 41 fps, Tmax-crush = 96
milliseconds. / 2) (Xmax-crush /
V). Accordingly, for Xmax-crush =
2.5 ft and V = 41 fps, Tmax-crush = 96
milliseconds. |
Now, with respect to the second
impact and the fact that Tmax-decel > Tmax-crush,
ITTE's research focussed on the right front seat,
which in those days was commonly called "the death
seat." That funereal phrase gave recognition to the
vulnerability of passengers seated thereon. A
post-crash photograph in the article showed
one hapless anthropometric dummy that suffered
horrendous consequences that day while riding in the
death seat. Let us continue
with a review of the 1954 analysis by ITTE's
research assistant...
| An
unrestrained passenger in the right front
seat would still be moving forward at 28 mph
= 41 fps while the vehicle abruptly
stops. The separation between the
passenger's head and the nearest structure
inside the car (ceiling, windshield,
dashboard) might be two feet. That
distance would be covered in about 50 ms,
which implied a shutter interval based on Tmax-decel = 96 + 50 =
146 ms. But there was more to
consider. |
| The anthropometric dummies had
"joints with movement and fixation closely
resembling that of a person forewarned of an
impending collision." With forewarning before the
first impact, a typical front-seat
passenger ought to be able to double that
50-ms delay, thereby deferring his or her second
impact to about 200 ms
after the car contacts the barrier. That was
the argument made by the research assistant
to the project engineer. |
And the rest is
history. Thus, the solution to the Historic Car Crash puzzle can
be...
|
The high-resolution
picture should be snapped 200 milliseconds
after the car contacts the barrier.
|
Epilog:
More than a half-century later,
sophisticated solvers will surely want to reconsider
-- all right, second-guess -- the three assumptions
above...
[1] Values of m and
k are surely not constant over the
range 0 < x < Xmax-crush.
Inasmuch as the harmonic period and therefore Tmax-crush is
proportional to (m / k)1/2, one must
expect that...
Tmax-crush will decrease with m,
as more and more parts of the vehicle -- especially
the engine -- become stopped against the
barrier.
Tmax-crush will increase as k
weakens during the crash with metals yielding and
fewer parts of the structure participating in the
'stiffness' of the 'spring'.
Thus these two effects seem to offset each
other, and thus did think ITTE's research assistant.
[2] Of course, something less than 100%
of the kinetic energy of the vehicle was absorbed by deformation
of that old Plymouth. For one thing, the back
end was lifted up in an obvious partial conversion of
kinetic to potential energy. Most likely, Ymax-crush acts in
reciprocity with Xmax-crush, but it is hardly clear, even today, what
effect energy shifts would have on Tmax-crush.
[3] Sophisticated solvers of the Historic
Car Crash puzzle undoubtedly doubt that a
perfectly linear average (f =Fmax-crush / 2) would
prevail throughout the range, 0 < x
< Xmax-crush. Today, with a simple spreadsheet,
people can lash up a piece-wise model to explore
non-linear elasticities for a crashing
automobile. However, many will be astonished to
see how little Tmax-crush changes with various shaped curves
postulated for k-versus-x.
|

 Featured in the Historic Car Crash puzzle is a
photograph taken by a Speed
Graphic camera like this one,
which dates back to the 1930s. Look closely and
you will see a solenoid
mounted horizontally below the lens. It was
designed to be activated by a speedgun
unit for synchronizing the shutter with a flash
attachment (not shown in the photograph).
Featured in the Historic Car Crash puzzle is a
photograph taken by a Speed
Graphic camera like this one,
which dates back to the 1930s. Look closely and
you will see a solenoid
mounted horizontally below the lens. It was
designed to be activated by a speedgun
unit for synchronizing the shutter with a flash
attachment (not shown in the photograph).