Magnanimous Month
Copyright ©2011by Paul Niquette. All rights reserved.

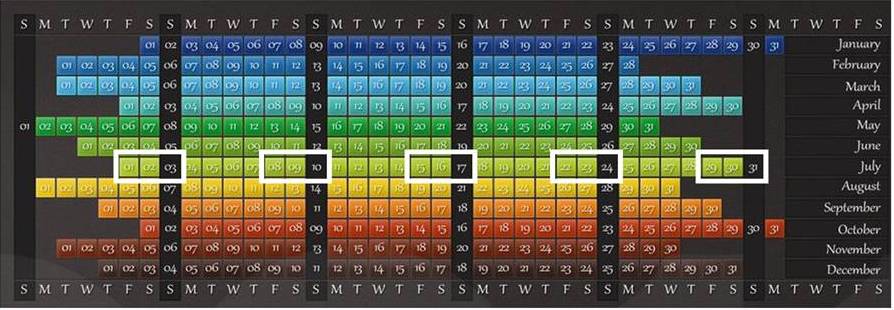
essages have been circulating throughout the worldwide web for years concerning a particular calendar event, proclaiming breathlessly that in 2011, July has five Fridays, five Saturdays. and five Sundays. "This happens once every 823 years!" exclaimed one version that reach a certain puzzle-master from an astounded correspondent. 

July, 2011

Sun
Mon
Tue
Wed
Thu
Fri
Sat
         
1
2
3
 4
 5
 6
 7
8
9
10
 11
 12
 13
 14
15
16
17
 18
 19
 20
 21
22
23
24
 25
 26
 27
 28
29
30
31
            

"Forward this message to your friends," says the message, "and money will arrive within four days, based on Chinese Feng Shui."

 How about a 'reward' for creating a puzzle out of that message?

Most of us take our weekends for granted, although their history dates back less than a century.  Both Saturday and Sunday have become welcome respites from the workweek.  Moreover, Friday is payday for many workers. The acronym TGIF has taken its place in the English Language as possibly the most sincere of many "Thank God" expressions.  Indeed, a restaurant franchise has trademarked T.G.I. Friday's no doubt as a positioning stratagem, appropriating a happy sentiment for the other six days. 
How pleasant to have payday followed immediately by two days off!  Let us define a Magnanimous Month as one in which those three consecutive days Fri-Sat-Sun occur five times. 
The year 2011 is depicted in what is called a Fractal Calendar below, showing the idiosyncratic behavior of months, weeks, and days in the Gregorian Calendar.  The five triads of Fri-Sat-Sun have been highlighted for the Magnanimous Month of July. Solvers are invited to confirm the following general observations:
  • Seven 'consecutive-day triads' are possible (Sun-Mon-Tue, Mon-Tue-Wed;...). 
  • Five identical 'consecutive-day triads' will fit exactly within a total of 31 days.
  • Seven months of every year have 31 days: January, March, May, July, August, October, December.
  • To qualify as a Magnanimous Month, its first day must be a Friday.


agnanimous Months should not be especially improbable events, as confirmed by snopes.com.  Suppose that the names of all 12 months have been written on slips of paper and mixed up in a bowl.  Taking a slip at random, the probability is 7/12 that a 31-day month has been selected.  The probability that that month begins on a Friday is 1/7, for a joint probability 7/12 x 1/7 = 1/12 = 0.0833 or 81/3 % that any month selected at random is a Magnanimous Month.

Thus, simple probabilities lead us to speculate that there is a Magnanimous Month about once per year. 

Sophisticated solvers are invited to estimate...
minimum and maximum times 

...between successive Magnanimous Months.

GO TO SOLUTION PAGE